Fluid Flow, Heat Transfer, and Mass Transport   Diffusion 

Diffusion, a Mass Transfer Phenomenon

Diffusion is a mass transfer phenomenon that causes the distribution of a chemical species to become more uniform in space as time passes.

In this case, species is a chemical dissolved in a solvent or a component in a gas mixture, such as the oxygen in air. The mass transfer of a species is the evolution of its concentration in space and time. If the concentration of a species is initially not uniform (the concentration might be greater in one region of a vessel than another, for example) then, over time, diffusion causes mass transfer in favor of a more uniform concentration.

The driving force for diffusion is the thermal motion of molecules. At temperatures above absolute zero, molecules are never at rest. Their kinetic energy means that they are always in motion, and when molecules collide with each other frequently, the direction of the motion becomes randomized. In most cases, these collisions are common; even in air at atmospheric pressure, which hardly seems a "dense" fluid, each molecule collides with a neighbor every few nanoseconds.

Understanding Diffusion: A Simple Example

When molecules are moving but also constantly changing direction, diffusion occurs because of the statistics of this movement.

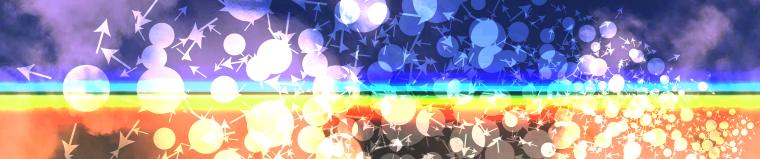
The image below shows a volume of a solution in which there is a nonuniform concentration. The red color indicates a high concentration of solute, whereas the blue color indicates nearly pure solvent.

After some time, diffusion causes the concentration in the vessel to become uniform:

In the figure below, the initial condition is shown with arrows whose size and direction show the number of molecules moving in a particular direction at one time – remember that their motion is random, so they'll be moving equally in all directions from any point:

At most points in the system, the uniform concentration means that the number of molecules moving in opposite directions is the same. As you can see, however, close to the boundary between the region of high and low concentration, there will be many more molecules moving to the right than moving to the left:

This is not because the molecules "prefer" to move in one direction, but just because there are more of them on one side of the boundary than the other. As a consequence, there is a net flux of material from left to right. This is diffusion.

In this case, mass moves from left to right so that the concentration becomes globally more uniform. Because diffusion drives a net flux of material from regions of high concentration to low concentration, we often speak of diffusion as acting "down a concentration gradient".

Once the concentration has become uniform, the molecules are all still in motion in different, random directions. However, there are now the same number of molecules moving across the boundary in either direction:

Even though the molecules are in random motion, there is no statistical driving force for them to start accumulating anywhere if their distribution is uniform. There is no net flux or change in concentration.

Laws of Diffusion

Although diffusion occurs because of statistical effects, when modeling diffusion, we normally use continuous partial differential equations (PDEs) to describe this statistical process. The relation of the above statistical process to the observed macroscopic phenomenon of "diffusion down a concentration gradient" was elucidated by Albert Einstein in one of his annus mirabilis papers of 1905 (3). There, he considered the related phenomenon of Brownian motion, i.e., the random motion of suspended particles like pollen grains.

The PDEs used to model diffusion problems might include Fick's laws, the convection-diffusion equation, or more complex methods for concentrated mixtures, like Maxwell-Stefan diffusion.

How Fast Is Diffusion?

Fick's laws contain only one parameter: the Diffusion Coefficient. This is the measure of the rate of the diffusion process.

Steady-State Diffusion

In a finite vessel with no sources or sinks of mass, the diffusion layer (where the concentration is nonuniform) eventually reaches the walls, after which a uniform, steady concentration will be attained. But in an infinite space or in the presence of a constant supply of material, a uniform concentration may not be attained. Compare the two animations below:

For finite vessels or sources, it is possible for a steady but nonuniform concentration to be attained. One example is diffusion to a disk at which mass is sunk. Here, a steady hemispherical concentration profile will arise after some time — as long as we keep supplying mass to the system. Tip: Read about the relation of dynamic and steady-state diffusion.

Published: January 14, 2015
Last modified: February 12, 2018