Technical Papers and Presentations

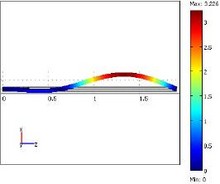
The present work formulates the dynamics of a slender structure as a long beam. This results in a PDE that is fourth-order in space, and second order in time. In COMSOL the coupling method is used to decompose this problem into two second-order equations, one for displacements, and one for bending moments, which are then solved to obtain frequencies and mode shapes.

For verification of approach, a simply supported uniform beam is considered, where the descriptive PDE’s are rendered non-dimensional in terms of scaled variables, and for a beam length of 1. COMSOL computation then results in a fundamental lowest-order frequency that exactly matches the analytical result of π².